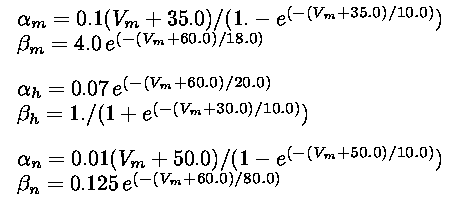The Hodgkin-Huxley Model Equations
In the 1950's Hodgkin and Huxley (1952) introduced the first continuum mathematical model designed to reproduce
membrane action potentials. In their model, they assumed that when the membrane potential was not equal to the equilibrium potential,
there would be a net flow of ions proportional to the difference between the membrane potential and the equilibrium potential.
Thus for an ion species labeled y with a corresponding current 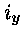 , Ohm's law gave the
equation
, Ohm's law gave the
equation 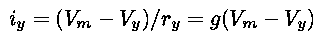 , where
, where 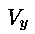 is the equilibrium potential,
is the equilibrium potential,  is the
resistance to the flow of ions, which can be expressed in terms of a membrane conductance
is the
resistance to the flow of ions, which can be expressed in terms of a membrane conductance 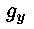 .
.
The ionic conductance is, in general, a nonlinear function of the membrane voltage and can be represented
by several channels. In cells, the ion channels are either open or closed, and in the case of voltage gating,
the percentage of channels that are open out of the total number of channels in the cell is a function of the
membrane voltage. Therefore, the probability function y (known as a gate variable) can be constructed to define
what fraction of the channels are open as a function of voltage and time. Since gate variables range between 0
(all ion channels closed) and 1 (all ion channels open), the total cell or tissue conductance gion due to a
given ion is given by 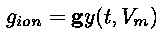 , where g is the maximum possible conductance obtained when all channels are open.
, where g is the maximum possible conductance obtained when all channels are open.
Hodgkin and Huxley found that squid axon sodium and potassium conductances, obtained from voltage-clamp
techniques, could be reproduced by using gate variables obeying simple first order equations of the form:
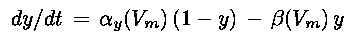 (Eq. 1)
(Eq. 1)
where  and
and 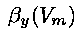 can be complex functions of the voltage. These equations describe how the gates
open and close at different time rates and as a function of voltage, thereby controlling the kinetics
of ionic flow through the channels.
can be complex functions of the voltage. These equations describe how the gates
open and close at different time rates and as a function of voltage, thereby controlling the kinetics
of ionic flow through the channels.
Hodgkin and Huxley reproduced the squid nerve action potential by using three different currents:
a potassium current  , a sodium current
, a sodium current 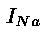 , and a leak current later identified as chlorine
, and a leak current later identified as chlorine
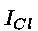 .
These three currents, whose sum is used to define the total ion current, are formulated as follows:
.
These three currents, whose sum is used to define the total ion current, are formulated as follows:
 (Eq. 2)
(Eq. 2)
Where h, m, and n are the gate variables and 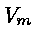 is the membrane voltage.
The opening and closing of the potassium ion channels is given
by the activation gate n , while the sodium channels are goverened by the activation gate m and the
inactivation gate h . In this model the sodium activation gate m operates on a time scale several
orders of magnitude faster than other gates. The functions
is the membrane voltage.
The opening and closing of the potassium ion channels is given
by the activation gate n , while the sodium channels are goverened by the activation gate m and the
inactivation gate h . In this model the sodium activation gate m operates on a time scale several
orders of magnitude faster than other gates. The functions  and
and 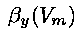 in this model take the following
form:
in this model take the following
form:
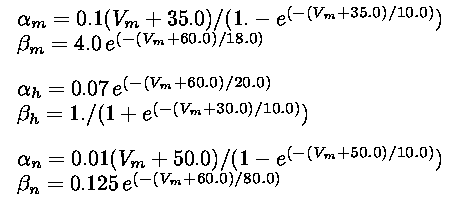
For further information about the model
we refer to the original article
J. Physiol. Vol 117 500-540, 1952 by A.L. Hodgkin and A.F. Huxley.
Back to the HH applet .
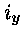 , Ohm's law gave the
equation
, Ohm's law gave the
equation 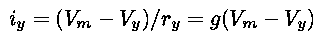 , where
, where 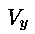 is the equilibrium potential,
is the equilibrium potential,  is the
resistance to the flow of ions, which can be expressed in terms of a membrane conductance
is the
resistance to the flow of ions, which can be expressed in terms of a membrane conductance 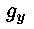 .
.
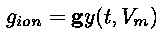 , where g is the maximum possible conductance obtained when all channels are open.
, where g is the maximum possible conductance obtained when all channels are open.
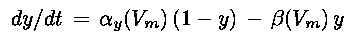 (Eq. 1)
(Eq. 1)
 and
and 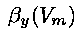 can be complex functions of the voltage. These equations describe how the gates
open and close at different time rates and as a function of voltage, thereby controlling the kinetics
of ionic flow through the channels.
can be complex functions of the voltage. These equations describe how the gates
open and close at different time rates and as a function of voltage, thereby controlling the kinetics
of ionic flow through the channels.
 , a sodium current
, a sodium current 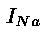 , and a leak current later identified as chlorine
, and a leak current later identified as chlorine
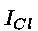 .
These three currents, whose sum is used to define the total ion current, are formulated as follows:
.
These three currents, whose sum is used to define the total ion current, are formulated as follows:
 (Eq. 2)
(Eq. 2)
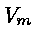 is the membrane voltage.
The opening and closing of the potassium ion channels is given
by the activation gate n , while the sodium channels are goverened by the activation gate m and the
inactivation gate h . In this model the sodium activation gate m operates on a time scale several
orders of magnitude faster than other gates. The functions
is the membrane voltage.
The opening and closing of the potassium ion channels is given
by the activation gate n , while the sodium channels are goverened by the activation gate m and the
inactivation gate h . In this model the sodium activation gate m operates on a time scale several
orders of magnitude faster than other gates. The functions